Plate Elements: Element Types

Description

Plate elements include the three and six-node triangular elements, and the four, eight and nine-node quadrilateral elements. The elements can be used for structural analysis of plane stress, plane strain, axisymmetric solids, plates, shells, shear panels and 3D membranes.

Element Types

Tri3

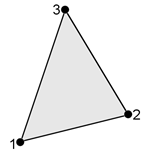
Quad4

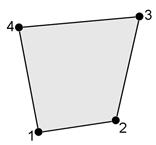
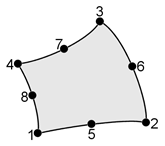
Tri6

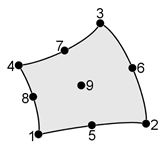
Quad8

Quad9

Analysis Types

2D Plane Stress

Plane stress analysis is used to model a thin, two-dimensional sheet of material. Plane stress assumes that all stresses are in the plane and the stress through the thickness is zero. Strain in the thickness direction can be non-zero. Plane stress elements must be defined on the global XY plane in Straus7. The only active degrees of freedom are those associated with displacement in the XY plane (i.e., DX and DY).

2D Plane Strain

Plane strain analysis is used to model the cross section of very thick or very long structures. Plane strain assumes that the strain normal to the plane is zero. Stress normal to the plane may be non-zero. Plane strain elements must be defined on the global XY plane in Straus7. The only active degrees of freedom are those associated with displacement in the XY plane (DX and DY).

Axisymmetric

A structure is said to be axisymmetric when it may be represented by a two dimensional section that can be extruded in a polar direction by 360 degrees about some axis to generate the 3D structure. It should be noted that in addition to geometric symmetry, the loading must also be symmetric around the axis symmetry. For example:

A point force applied to an axisymmetric node is taken as a per radian value.
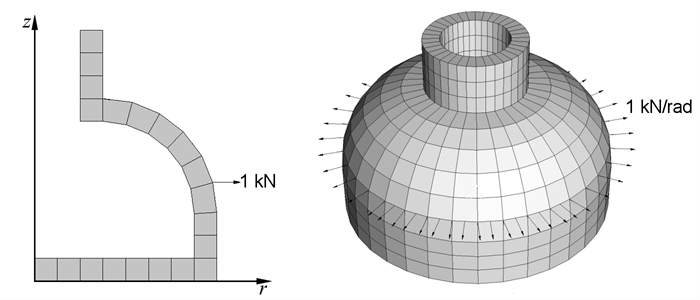
Pressures applied to axisymmetric edges are equivalent to all-around pressure in the full model.

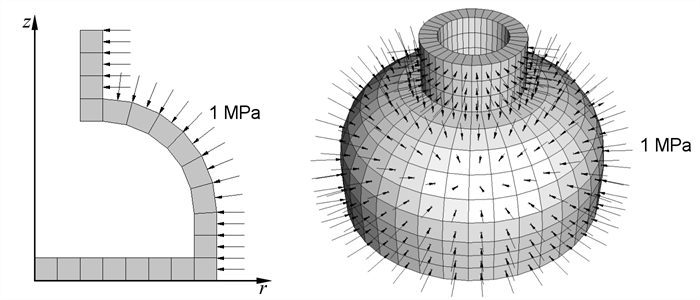
In Straus7, axisymmetric elements must be positioned on the global XY plane with all X coordinates greater than or equal to zero. The Y axis is assumed to be the axis of rotational symmetry . The X axis is the radial direction .

The only active degrees of freedom are those associated with displacement in the XY plane (DX and DY).

Plate/Shell

The plate/shell element is the most general type of plate element. The element permits in-plane and out-of-plane displacements. Plate/shell elements may be used for the analysis of flat plates and general three-dimensional shells.

Tri3
A flat facet element formulated by combining membrane action and bending action. The membrane part of the element models a state of constant strain. The bending part is based on the so-called DKT (Discrete Kirchhoff Triangle) formulation for thin shells.
Quad4
A linear element that is based on the so-called thin shell theory and formulated as a DKQ (Discrete Kirchhoff Quadrilateral). The element is a high performance element when modelling thin shells.
Tri6, Quad8, Quad9
A suite of quadratic elements from the Lagrange family that are based on the thick shell theory. The mid-side nodes offer additional flexibility in modelling curved edges and curved surfaces. Mid-side nodes should be positioned near the physical mid-point between the corner nodes and never outside the middle half of the edge for the mapping between physical space and parametric space to be valid. As these elements are curved, there will be limitation on the thickness compared with their curvature. Straus7 issues relevant warnings when the thickness if too large for the curvature. The Tri6 version of the element is obtained by collapsing one edge (i.e., three nodes) onto a single node.

Shear Panel

This is a specialised element available only as a four-node quadrilateral. The element carries only in-plane shear forces along the edges. It is commonly used in the modelling of transport vehicles that have semi monocoque structures composed of stringers and thin sheet such as aircraft structures, or sheet cladding on structures. Such cladding or thin sheet is not capable of sustaining in-plane compression loads because it buckles easily but it can sustain large shear forces and tensile forces.

3D Membrane

The 3D membrane is a plate element that has in-plane (membrane) stiffness only. That is, it can carry direct stress and in-plane shear stress. It has no bending stiffness. It is basically a 3D version of the plane stress element, in that it is not restricted to the XY plane. The 3D membrane element is typically used for geometric nonlinear analysis. The type of structures modelled with this element typically undergo large deflections and develop significant membrane stresses to support the applied loads. Examples include elastic membrane balloon type structures and fabric roofs.